Aly等人2019年论文《Efficient Symmetric Primitives for Advanced Cryptographic Protocols (A Marvellous Contribution)》。
sponge结构用于构建hash函数 from an underlying permutation by iteratively applying it to a large state。该state包含 b = r + c b=r+c b=r+c位,其中:
- r r r:为sponge rate
- c c c:为sponge capacity
可将长度变化的input,通过在每次递归过程中absorb input中的 r r r bit,hash为任意长度的output。
2. Rijndael-128 block cipherRijndael-128 cipher,俗称AES-128,包含了5个基础块:
- AddRoundKey
- SubBytes
- MixColumns
- ShiftRows
- ExpandKey(其中包含SubWords、AddWords、RotWords和AddConstants)
本文主要关注S-Boxes的调整(即SubBytes和SubWords)。 每个S-Box以一个字节为单位进行处理,S-Box ( z ) = g ( f ( z ) ) (z)=g(f(z)) (z)=g(f(z)),其中:
- f f f 函数(求倒数函数): f : F 2 8 → F 2 8 : x ↦ x 254 f:\mathbb{F}_{2^8}\rightarrow \mathbb{F}_{2^8}:x\mapsto x^{254} f:F28→F28:x↦x254,为adapted multiplicative inverse function over F 2 8 \mathbb{F}_{2^8} F28。可抵抗differential攻击和linear攻击,同时可支持与MixColumns和ShiftRows扩散函数一起用于wide trail design strategy。【注意,有限域内有: x p − 1 m o d p ≡ 1 x^{p-1} \mod p\equiv 1 xp−1modp≡1,从而有倒数运算: x − 1 = x p − 2 x^{-1}=x^{p-2} x−1=xp−2。】
- g g g 函数(affine多项式): g : F 2 8 → F 2 8 : x ↦ M x + b g:\mathbb{F}_{2}^8\rightarrow \mathbb{F}_2^8: x\mapsto Mx+b g:F28→F28:x↦Mx+b,为 SubBytes step 中的affine transformation。其中 M ∈ F 2 8 × 8 , b ∈ F 2 8 M\in\mathbb{F}_2^{8\times 8}, b\in \mathbb{F}_2^8 M∈F28×8,b∈F28。这种转换有助于增加S-Box结果的复杂性,以抵抗algebraic 攻击。 当affine transformation针对 F 2 \mathbb{F}_{2} F2时,则整个S-Box可 以如下多项式表示(over F 2 8 \mathbb{F}_{2^8} F28): S-Box ( z ) = 0 x 05 ⋅ z 254 + 0 x 09 ⋅ z 253 + 0 x F 9 ⋅ z 251 + 0 x 25 ⋅ z 247 + 0 x F 4 ⋅ z 239 + 0 x 01 ⋅ z 223 + 0 x B 5 ⋅ z 191 + 0 x 8 F ⋅ z 127 + 0 x 63 (z) =0x05 \cdot z^{254}+0x09\cdot z^{253}+0xF9\cdot z^{251}+0x25\cdot z^{247}+0xF4\cdot z^{239}+0x01\cdot z^{223}+ 0xB5\cdot z^{191} +0x8F\cdot z^{127}+0x63 (z)=0x05⋅z254+0x09⋅z253+0xF9⋅z251+0x25⋅z247+0xF4⋅z239+0x01⋅z223+0xB5⋅z191+0x8F⋅z127+0x63
MiMc的构建方式有:
- 采用Albrecht等人2016年论文《Mimc: Efficient encryption and cryptographic hashing with minimal multiplicative complexity》中的block cipher构建,表示为MiMC-q/q:(其中
q
q
q为prime或为 prime power of 2。)在每一个round,每个state仅包含一个field element,包含一个field element in
F
\mathbb{F}
F 会做立方运算,再与随机常量
C
i
C_i
Ci相加,每一个round插入的key
K
K
K是完全相同的。对于128位状态,需要82 rounds就足够。

- 采用Feistel network构建,表示为MiMC-2p/p:(其中
p
p
p为prime或为 prime power of 2。)在每一个round,每个state包含2个
F
p
\mathbb{F}_p
Fp elements,分别表示为
x
L
x_L
xL和
x
R
x_R
xR。第
l
l
l round的函数为:
x
L
∣
∣
x
R
←
x
R
+
(
x
L
+
K
+
C
l
)
3
∣
∣
x
L
x_L||x_R\leftarrow x_R+(x_L+K+C_l)^3||x_L
xL∣∣xR←xR+(xL+K+Cl)3∣∣xL 其中
K
K
K为key,
c
l
c_l
cl为第
l
l
l round常量。对于128位状态,需164 rounds就足够。

采用Rijndael-128 block cipher,针对的field为 F 2 n / m m \mathbb{F}_{2^{n/m}}^{m} F2n/mm,具有 n n n-bit staten、 n n n-bit key和 n n n bits security。 Vision的最小round数为10,推荐round数为 2 ⌈ n / 5.5 m ⌉ 2\left \lceil n/5.5m \right \rceil 2⌈n/5.5m⌉。 Vision中:
-
f
f
f 函数(求倒数函数)为:
 g
g
g 函数(affine多项式)为:奇数步为
B
−
1
(
x
)
B^{-1}(x)
B−1(x),偶数步为
B
(
x
)
B(x)
B(x)。
g
g
g 函数(affine多项式)为:奇数步为
B
−
1
(
x
)
B^{-1}(x)
B−1(x),偶数步为
B
(
x
)
B(x)
B(x)。 

key schedule:是指基于一个key派生出所有round keys的算法。(calculates all the round keys from the key)。 Vision的key schedule为: 
详细的Vision算法实现为: 
与Vision类似,但是其针对的域为 F p \mathbb{F}_p Fp,而不是 F 2 n / m \mathbb{F}_{2^{n/m}} F2n/m。 Rescue的最小round数为10,推荐round数为 2 ⌈ s / 4 m ⌉ 2\left \lceil s/4m \right \rceil 2⌈s/4m⌉,其中 s s s为security level。 其中:
- inverse power map为:(大多数域,选
α
=
3
\alpha=3
α=3就足够,但是对于
2
255
−
19
2^{255}-19
2255−19,不满足
g
c
d
(
p
−
1
,
3
)
=
1
gcd(p-1,3)=1
gcd(p−1,3)=1,可选择
α
=
5
\alpha=5
α=5。对应的security level为
log
2
(
p
m
)
\log_{2}(p^m)
log2(pm) bits,即
m
m
m 乘以
p
p
p的二进制表示位数。)

Rescue over
F
p
m
\mathbb{F}_p^m
Fpm carries
m
m
m field element as its state which goes through a nonlinear operation, an MDS matrix
M
M
M, and a key injection in each step。 Rescue中每一个round分为2个step:  详细的Rescue算法实现为:
详细的Rescue算法实现为: 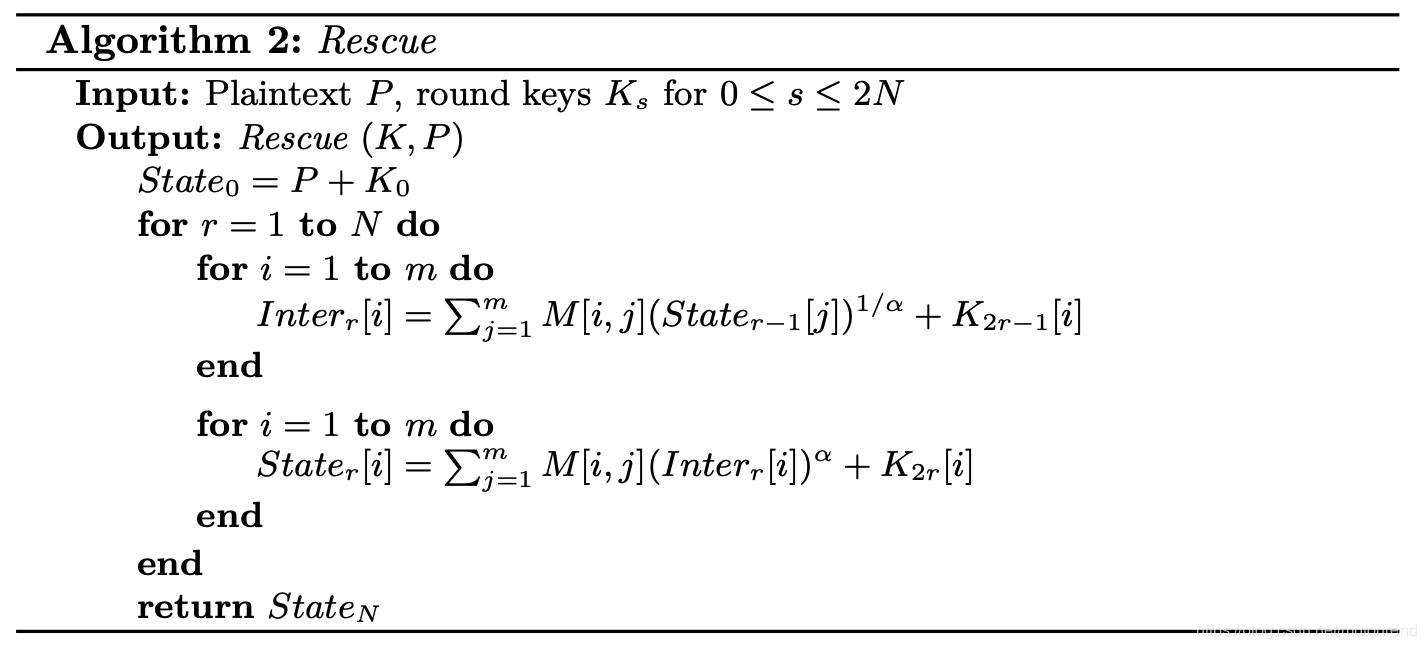
Rescue的key schedule为: 
Algebraic Intermediate Representation (AIR) constraints。 接下来对比将Vision、Rescue、MiMC表示为AIR constraints的性能: 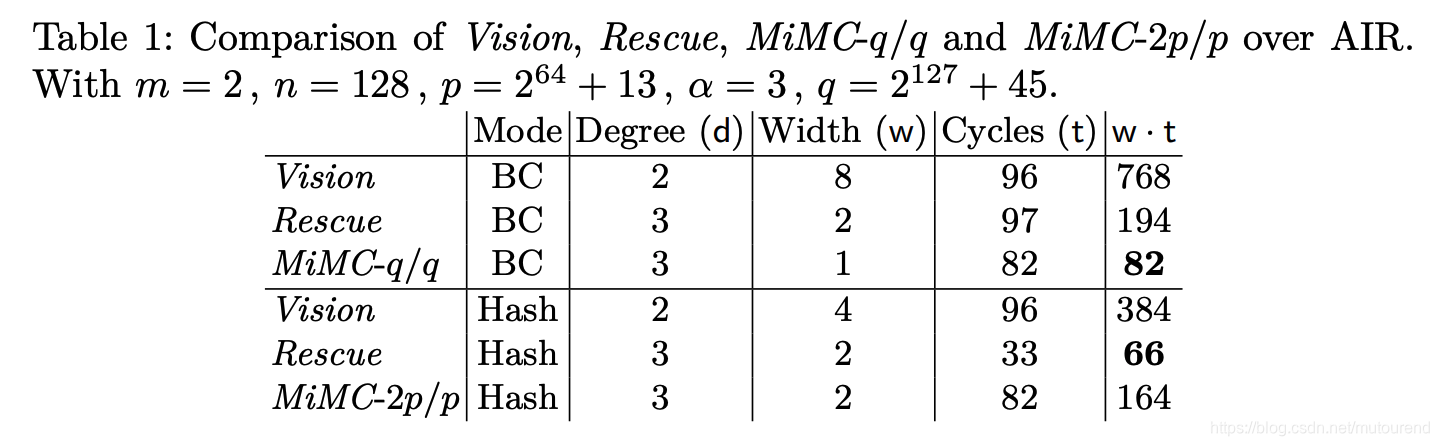
对比将Vision、Rescue、MiMC表示为R1CS的性能: 
借助masked operation将Vision、Rescue、MiMC用于MPC,相应的性能对比为: 



